The only reason people make investments is to get returns.
Most people think that they know how to calculate actual returns. But many don’t use the right method to calculate returns. Using the wrong method to calculate returns can give an incorrect picture of your investments.
For example, a person tells you that his investments of Rs 25 lacs in stocks are now worth Rs 75 lac. He is very happy to have tripled his original investment. But when he tells you that this increase has taken place in 15 years, will you still consider it to be a good investment?
We try to answer this question by understanding the 3 main ways to calculate returns
- Absolute returns,
- Compounded annual growth rates &
- IRR
Let’s use the above example to understand these methods.
Absolute Returns
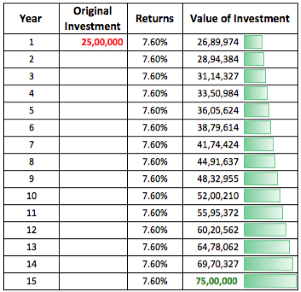
So in our example, Initial Investment = Rs 25 lac, Final Value of Investment = Rs 75 lac & Time Period = 15 years
- (Rs 75,00,000 – Rs 25,00,000)/Rs 25,00,000 in %
- Rs 50,00,000 / Rs 25,00,000 in %
- 200% (which means 3 times)
As you must have noticed, the calculation of absolute returns does not consider the time period. Absolute Return is the simplest way of calculating returns. But since it doesn’t consider the time period of returns, its utility is extremely low when you need to compare returns of various investments.
A better option to calculate returns is CAGR.
CAGR
CAGR stands for Compounded Annual Growth Rate. It is the average annual growth rate of an investment over a specified period of time. CAGR is calculated using the following formula
So in our example earlier, CAGR will be calculated as: ((Rs 75 lac / Rs 25 lac)^(1/15)) -1 = 7.6%
This doesn’t seem like a great return now. Isn’t it?
- This is the reason why CAGR is used to calculate returns as it gives a more accurate picture of returns earned.
- To understand how a CAGR of 7.6% increases the value of the original investment of Rs 25 lac, please have a look at the table below:
CAGR assumes that returns are constant and investment will be growing at a constant rate.
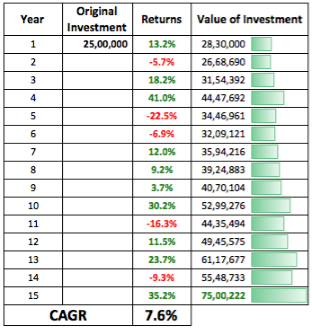
Now as you might have noticed, CAGR is a theoretically calculated return figure that determines a fixed annual growth rate on an investment, which will result in the final investment value. In reality, it doesn’t happen like this – at least in stock market-related investments. Returns can vary from one year to other. It can, in fact, be something like this:
So as you see in the table above, the returns vary a lot every year. But CAGR smooth-ens out the volatility in returns and tells you one figure (7.6%), which would have produced the final (required) investment value, if returns were equal to the calculated figure (of 7.6% in our example) every year. To bring more clarity to the concept of CAGR, try to understand this that just because a financial investment has given 7.6% returns on average (CAGR), it doesn’t mean that the investment would have actually grown by 7.6% every year.
IRR
Internal Rate of Return is used to calculate returns when you are investing some amount at fixed intervals. So this method of return calculation is suitable for those people who invest/save on a periodic basis. The only condition to use this method is that investment should be made at regular time intervals. It can be weekly, monthly or even annually.
Let us see how it is done.
For simplicity, we will take an example where investments are made once every year. So suppose you invest Rs 12,000 every year for 15 years. After 15 years of annual investments, the value of your corpus is Rs 6.5 lac. How will you calculate the returns then?
You simply cannot compare your total investments of Rs 1.8 lac (15 x Rs 12,000) with the final value as each investment has happened in a different year. It is here that IRR comes to the picture. MS Excel function can be used to calculate IRR. In our example, the IRR comes out to be 14.89%. And this is how you can visualize it
(Note in excel, while using the formula for IRR, the investments, i.e. outflows are taken as -ve whereas inflows are taken as +ve).
So as you see, there are several ways of calculating returns. Each one is suitable for different cases.
As investors, its very important to understand how to calculate the actual returns. And that is because if you know the real returns, only then you will be able to compare different investment options and take the right investment decision.